In case anyone is curious about the PHA tuning adjustments for the above high speed maps I'll remind everyone that when utilizing a large range of count rates we should keep in mind the pulse height depression which occurs at high count rates.
This pulse height depression effect causes the PHA peak to shift towards lower voltages at higher count rates, thus increasing the possibility of some counts being cut off by the baseline level at these higher count rates. The solution is to always tune ones PHA settings at the highest expected count rate (highest beam current on a material with the highest expected concentration- usually one's primary standard).
In the above high speed mapping example we utilized SiO2 as the primary standard for Si Ka, therefore we should tune our PHA settings on that material at the highest expected beam current. However, since we intend to acquire our olivine unknowns at 200 nA and our primary standards at only 30 nA, and since the concentration of Si in SiO2 is about 50% and the concentration of Si in olivines is about 20% (in round numbers), we could compare these concentrations and beam currents by considering that our olivines have 2.5 times less Si than our primary standard, but will be measured at 6.6 times the beam current, so we should probably tune our Si Ka PHA on the olivine unknown at 200 nA for the highest expected count rate (of course the exact count rate depends on the absorption correction differences between these materials also, but we're just speaking in round numbers here).
But to make things more interesting I decided to tune the PHA settings on the primary standards at 200 nA. Here is Si Ka on SiO2 at 200 nA:
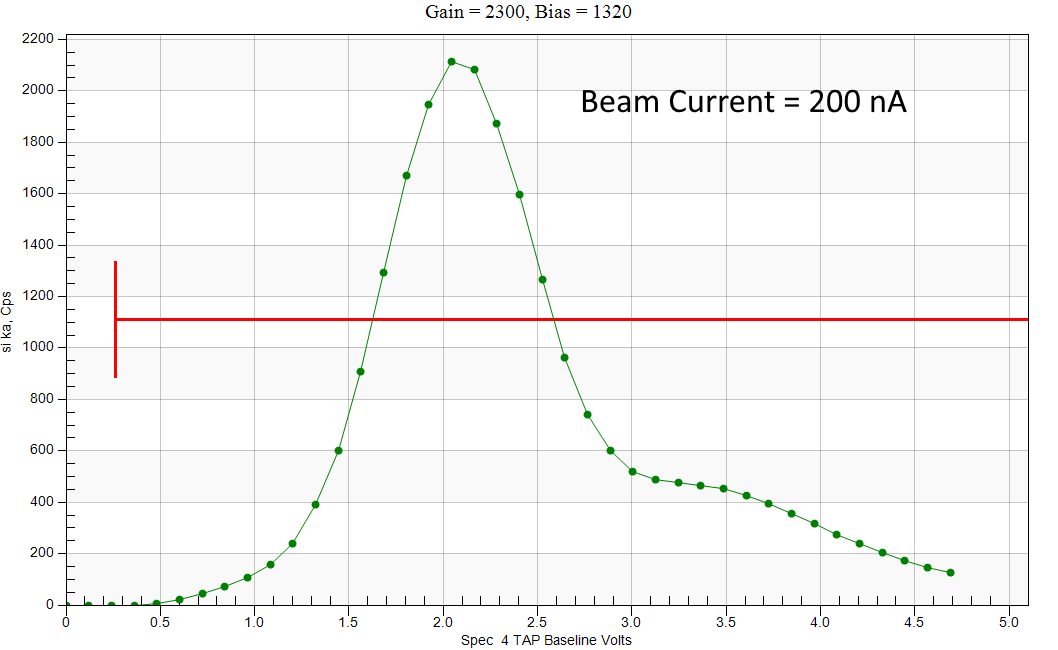
Note that the gain was adjusted to place the PHA peak fully above the baseline level even at this quite high count rate (~160 kcps). And remember, although the PHA peak appears to be slightly cut off at the right side of the plot, that is merely an artifacts of the PHA display system. All counts to the right of the plot axis are fully counted because we are in INTEGRAL mode.
Next here is the Si Ka PHA scan again on SiO2 at the same PHA settings but using a beam current of 30 nA:
We can see that the PHA peak has shifted even further to the right, but again we don't care as all pulse to the right of the plot will all be counted in INTEGRAL mode.
Remember, on Cameca instruments we will be adjusting the gain to place the PHA peak above the baseline at the highest expected count rate, while on JEOL instruments, we will be adjusting the bias the place the PHA peak above the baseline level at the highest expected count rate.
We are a community of analysts, that cares about EPMA